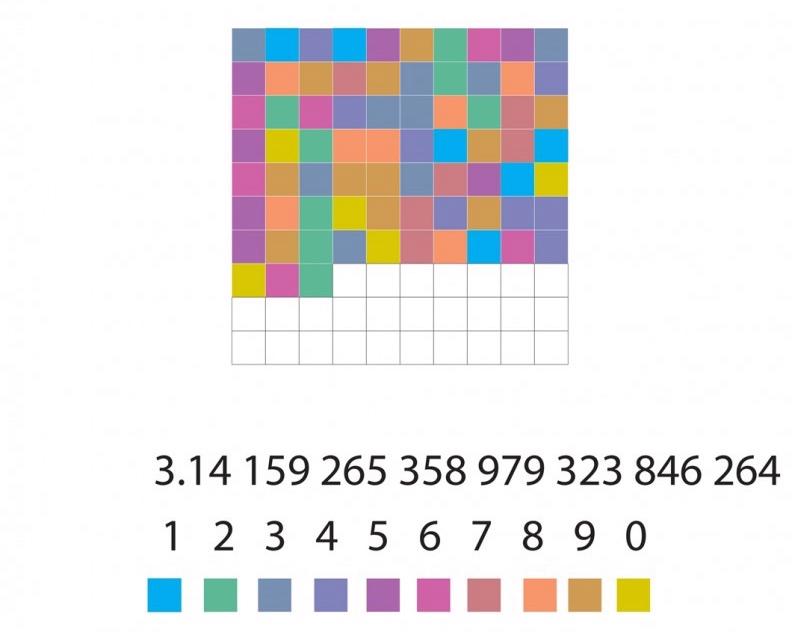
There are many things you can do with pi. You can use it to calculate the rotor circumference of the world’s most powerful offshore wind turbine in operation. It equals a whopping 691 meters, or 0.43 miles! Or do the same thing for the world’s largest jet engine. The circumference of its front fan clocks in at 10.52 meters, roughly equivalent to a tower of 50 carry-ons stacked on top of one another. You can also paint it. “Pi fascinates me,” says artist Stewart Kenneth Moore. “I thought, if I take all these numbers and express them as colors, will something emerge?”
Pi, of course, is the world’s most famous irrational number. This ratio between the circumference and the diameter of a circle is always the same — we generally round it to 3.14, which is why Pi Day falls on March 14. Yet no one has seen pi in its full glory because the numbers behind the decimal point run into infinity. “That’s the reason why we represent the number by a symbol, the Greek letter π,” says Jim Bray, chief scientist at GE Global Research. “This number is not just interesting, but it’s also very important. Many of the engineering formulas that we use to design jet engines or turbines for generating electricity contain π.”
Humans have known about pi since they started using wheels 4,000 years ago. Ancient Babylonians could celebrate an entire “Pi Month” because they rounded pi down to 3 — as did the authors of the Old Testament.
In 300 B.C., Euclid, the “father or geometry,” opened a path for calculating pi by looking at the circle as a polygon with infinite sides. Archimedes, one of antiquity’s greatest mathematicians, applied Euclid’s theorems to arrive at pi a few decades later. He also paid for it with his life. When Roman soldiers occupying his hometown, Syracuse, walked over his math drawings in the sand, he told them to get lost. His apocryphal last words? “Do not disturb my circles.”
Today we know pi to 12 trillion digits, but the calculation can’t be completed. That’s why some artists decided to tackle pi from a different angle. One team has turned it into music. Scottish painter Stewart Kenneth Moore assigned colors to numbers from 0 to 9 and painted it. He most recently painstakingly painted pi to 1,521 decimal places, one colorful pixel after another.
We talked to Moore, who signs his work as Booda, on the eve of Pi Day, which also happens to be Albert Einstein’s birthday. Here’s an edited version of our conversation.
GE Reports: What draws you to pi?
Stewart Kenneth Moore: It’s endless. The infinite quality of it, the lack of repetition — it fascinates me. I thought, if I take all these numbers and express them as colors, will something emerge? I was thinking about pareidolia, this thing that happens when you look at a cloud and you think you see a face. It's not there, but it is in your mind. I was wondering if the jumble of pi decimals on a wall expressed as colors would maybe generate things in the minds of the people that looked at them.
GER: By putting your work online, you are harnessing the collective hive mind by asking people to help you catch a glimpse of pi?
SKM: That’s another way to look for meaning. We’ve evolved over thousands of years to stare at people and read facial expressions for signs of danger and intent. What is the person I'm facing thinking, or what is the animal I'm looking at planning to do? We all have got this overactive tool searching for expressions and meanings and I just wondered if there was anything that would emerge from staring at a mass of colors.
GER: Have you seen anything?
SKM: The problem with pi is that it doesn't repeat too much, so there's nothing really to keep your eye. But the painting can become three-dimensional. The colors seem to be jumping back and forth. Certain squares seem to come forward, and others seem to slip back. It's a little like looking down on buildings in Manhattan.
GER: Is there any repetition?
SKM: There is a sequence of six 9s at the 762nd decimal place. When mathematicians first got there, they must have thought they were done. That's it, it's over! But pi then kicks off again. I always think that the band of nines is really going to dominate in the painting. Then I stand back and it just vanishes into the whole thing.
GER: When did you become interested in pi?
SKM: I always wanted to paint math. I believe there must be a better way to teach mathematics, and I decided to use colors to make it accessible. In the 1990s, I got a book about pi. It was fascinating to me. I also do graphic design, and I often work with pantones, the little swatches of color. When I looked at the numbers, I immediately saw them turn into colors.

GER: How do you start your pi paintings?
SKM: I draw a grid on a blank square canvas and fill in the numbers — 3, 1, 4, 1, 5 and so on. Then I pick 10 colors. For the pi painting I just finished, the selection was totally random. I just pulled the paints out of the box and said, “that one, this one, that one.” Then I proceed in filling out all the 1s, then the 2s and so on. In the beginning it goes very fast, but very quickly you start to box yourself in.
GER: Why?
SKM: You have to be very careful with the edges because you have to wait for the previous numbers to dry. You don't want the paint to start mixing. The 9s and zeros, which I paint last, always take several days to add. Then I wait for a month or two, and once it's fully dry, I varnish it, frame it and hang it. That's the process. It's an oil painting.
GER: What have you discovered so far?
SKM: Usually when you start a picture, you've got an idea of what you're going to do. Still, some artists will start a picture with no idea of what is going to happen. This is the best of both worlds because you start with an idea and you know the structure — one is one, two is two — but you don't know the result. My latest pi painting represents 1,521 decimal places. I can't possibly imagine what all those randomly chosen colors are going to do at this scale when they're together. You know what you're doing, but you have no idea what the outcome will be. That's really fascinating to me.





